The host system of the cuprate HTSC is an antiferromagnet. With a small percentage of doping the AF order rapidly disappears, and the system evolves into a spin liquid. Recent experiments are observing traces of various spatial structures such as charge stripes, in the vicinity of this area in the phase diagram. Scanning tunnel microscopy has also revealed some intrinsic nonhomogeniety on intermediate scales in these systems.
Then what are the relations, if any, between these structures, their magnetic excitations, and the emergence of superconductivity? Rather than plunging directly into the debate on what the precise describtion of the pseudogap phase should be, our choice was to begin from a well defined spin state, a spin Peierls system, which is a spin singlet state with confined S=1/2 spinons, and the simplest nontrivial spatial structure.
As a first step, we have devised a bosonzation scheme which enables us to incorporate the powerful machinery of abelian bosonization, but at the same time taking into account the full effect of the directional fluctuations of the staggered magnetization. We find under certain conditions, that in hole-doped quasi-1d spin-Peierls systems, strong enhancement of singlet superconductivity results from quantum mechanical phase-interference effects between the holes and the antiferromagnetic background (Fig1); basically, the holes will revive the spectral weight of the AF fluctuation within its local neighborhood, and these AF fluctuations will in turn enhance the coherent charge transport and mediate interaction between the holes. We are now extending our investigations to 2d systems (Fig2).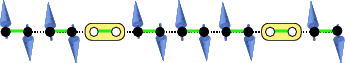
Fig.1: pairing of holes in quasi-1d spin-Peierls system
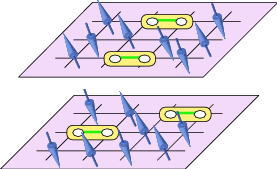
Fig.2 : 2d system